|
Some
Common Themes in
Robert W. Fathauer Tessellations Company Tempe, AZ 85281, USA E-mail: tessella@futureone.com
Abstract
Historically, mathematics has played an important role in visual art, particularly in perspective drawing; i.e., the means by which a three-dimensional scene is rendered convincingly on a flat canvas or piece of paper. Mathematics and art are two seemingly disparate fields according to contemporary views, the first often considered analytical and the second emotional. Mathematics does not play an overt role in most contemporary art, and in fact, many artists seldom or never employ even perspective drawing. However, there are a number of contemporary visual artists who make mathematics a focus of their work. Several notable figures in history paved the way for these individuals. There are obviously no rules or limits on themes and ideas in mathematical art. However, there are a number of themes that have been widely used by mathematical artists. Some of these are described here, with examples. These themes include polyhedra, tessellations, impossible figures, Möbius bands, distorted or unusual perspective systems, and fractals. This paper is intended to
some degree to serve as an introduction to the Exhibit of Visual Mathematical
Art held as part of Bridges 2001. The examples given here are taken for
the authorís convenience from a limited set of artists. The choice of works
is by no means intended to be a representative sampling of artists working
in this area.
2. Notable figures in the history of mathematical visual art The Dutch graphic artist M. C. Escher (1898-1972) is in some ways the father of mathematical art. Mathematical ideas play a central role in most of his art, with the exception of his early work. Most of the themes commonly employed by contemporary mathematical artists were also explored by Escher, and his work is clearly a major source of inspiration for many contemporary artists. It is assumed that the reader is familiar with Escherís work, which can be explored in depth in the books cited in references 1-3. A number of other notable figures who are not as commonly associated with mathematical art are described in this section. One of the common themes in mathematical art is polyhedra. These have been studied from very early on. Plato (427-348 BC) wrote about the five regular solids commonly known as the "Platonic solids". However, these predate him, and details concerning the original discovery of these polyhedra are not known. Plato related these solids to the basic elements, with fire assigned to the tetrahedron, air to the octahedron, water to the icosahedron, and earth to the cube. Plato further wrote: "There was yet a fifth combination which God used in the delineation of the Universe" (Timaeus, 55d-e), namely the dodecahedron. Archimedes (c 290/280-212/211 BC) described the 13 semi-regular polyhedra (see below). Just as the regular polyhedra are referred to as the Platonic solids, the semi-regular polyhedra are commonly referred to as the Archimedean solids. His writings on them were lost, together with the figures. They were gradually rediscovered during the Renaissance, and all 13 did not appear together until Johannes Kepler's Harmonices Mundi was published in 1619, nearly two millennia after Archimedes' death. Leonardo da Vinci (1452-1519) is famous for his accomplishments both as an inventor and as an artist. His notebooks contain the first known examples of anamorphic art, which employs distorted perspective grids. These "slant anamorphoses" present objects that must be viewed at an angle to appear undistorted. Johannes Kepler (1580-1630), best known for his work in astronomy, also had a keen interest in geometric tessellations and polyhedra. In his book Harmonices Mundi (1619), he published a number of tilings of regular and star polygons, in addition to the polyhedra mentioned above. Koloman Moser (1868-1918) was a graphic artist who taught in Vienna and worked in the Art Nouveau style. He executed a couple of tessellations with fish motifs around 1899-1900 that are remarkably Escheresque in style, though Escher was apparently not aware of Moser's work until 1964. Some of the twentieth centuryís best-known artists drew heavily on mathematics. Piet Mondriaan (1872-1944) was a Dutch painter known for his geometric abstractions; several of his paintings depict rectangular blocks of color separated by black lines. Salvador Dali (1904-1989) was a flamboyant and controversial Spanish Surrealist painter who employed mathematics in some of his work. His "Crucifixion" of 1954 depicts a hypercube, and his "La Visage de la Guerre" of 1940 depicts a fractal progression of ever smaller grotesque visages. He also executed a set of erotic anamorphic paintings. René Magritte (1898-1967) was a Surrealist painter who often played games with perception. For example, one of his paintings blurs the line between what is painted on a canvas sitting in front of a window and the view outside the window. Max Bill (1908-1994) was a graphic artist, sculptor, and painter who studied at the Bauhaus. He made a number of sculptures based Möbius strips, many of which are displayed in public places. Victor Vasarely (1908-1997) was a Hungarian-born painter known as the leading pioneer and practitioner of Optical Art ("Op Art"). He used the coloring of simple geometric shapes, often in arrays, to suggest motion and concave/convex effects on a flat canvas. Benoit Mandelbrot
(1924-) is the mathematician largely responsible for formalizing and popularizing
the concept of fractals. He discovered the Mandelbrot set, the best known
of fractal objects. He also coined the term "fractal", derived from the
Latin word "fractus", meaning fragmented or broken. His recognition of
the esthetic content of fractals is shown in the following quote. "Can
pure geometry be perceived by the 'man in the street' as beautiful? To
be more specific, can a shape that is defined by a simple equation or a
simple rule of construction be perceived by people other than geometers
as having esthetic value - namely, as being at least surprisingly decorative
- or perhaps even as being a work of art? When the geometric shape is a
fractal, the answer is yes." [4]
3. Common themes in mathematical visual art A number of themes are commonly found in mathematical visual art. These include polyhedra, tessellations, impossible figures, Möbius bands, distorted or unusual perspective systems, and fractals. Individual mathematical artworks often combine more than one of these themes. Each of these themes is described below, with examples of artworks that employ them. A. Polyhedra A polyhedron is a three-dimensional
solid, the faces of which are polygons. There are only five convex polyhedra
for which each face is the same regular polygon and all vertices are of
the same type. These are known as the Platonic polyhedra, or Platonic solids.
There are thirteen convex polyhedra for which each face is one of two or
three different regular polygons, and for which all vertices are of the
same type. These are known as the Archimedean solids. In addition, there
are an infinite number of facially regular prisms and antiprisms. Escher
employed polyhedra in a number of his prints, including "Reptiles" (1943),
"Double Planetoid" (1949), and "Gravity" (1952) [1].
An example is shown below of a contemporary work by Dick Termes.
B. Tessellations A tessellation, also known
as a tiling, is a collection of shapes that fit together without overlaps
or gaps to cover the mathematical plane. A regular tessellation is one
made up of regular polygons that are all of the same type, and for which
all vertexes are of the same type. There are only three regular polygons
that tessellate in this fashion: equilateral triangles, squares, and regular
hexagons. A semi-regular tessellation is one made up of two or three different
types of regular polygons, and for which all vertexes are of the same type.
There are eight semi-regular tessellations. The three regular and eight
semi-regular tessellations are collectively known as the Archimedean tessellations.
Tessellations in which the individual tiles are recognizable motifs is
a topic with which Escher was fascinated for most of his career. He kept
notebooks in which he enumerated over 130 such designs [3].
He employed these in a large fraction of his prints, including "Day and
Night" (1938), his "Circle Limit" prints I-IV (1958-1960), and his "Metamorphosis"
prints I-III (1937-1968). Examples are shown below of contemporary works
by Hollister David and Robert Fathauer.
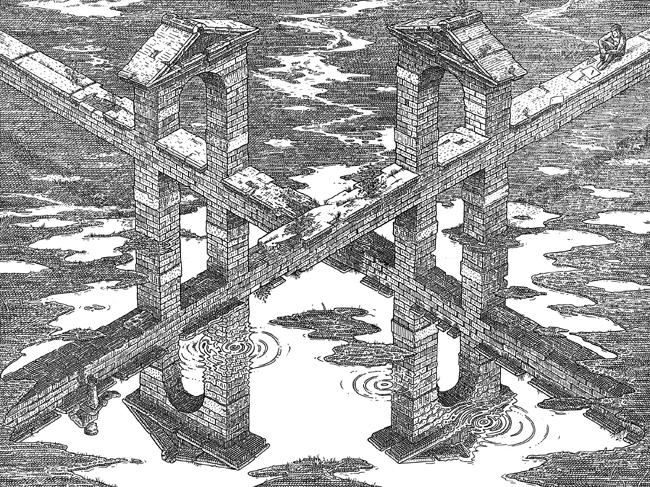
C. Impossible figures An impossible figure is one
that is depicted in a perspective drawing in such a manner as to appear
normal at first glance. However, on closer examination, the viewer finds
that such an object could not exist in the three-dimensional world. Escher
employed such figures in some of his most popular prints, including "Belvedere"
(1958), "Ascending and Descending" (1960), and "Waterfall" (1961). An example
is shown below of a contemporary work by István Orosz.
D. Möbius bands and other abstract surfaces A Möbius band is a one-sided object. This can be easily constructed by taking a strip of paper, giving it a half twist, and then taping the two ends together. Escher employed Möbius bands in "Horsemen" (1946), "Möbius Strip II (Red Ants)" (1963), and "Knots" (1965). More recently, minimum energy
surfaces have been a source of inspiration for mathematical artists. For
example, a minimum energy surface is the sort of surface a soap film forms
when bridging a wire frame of some shape. Brent Collins, whose work is
well known to Bridges attendees, uses Möbius bands, minimum energy
surfaces, and other variations of ribbon-like abstractions in his sculpture.
E. Distorted or unusual perspective systems Unusual perspective systems, those with other than the usual one, two or three vanishing-point systems are another common source of inspiration. A related device is anamorphic art. Escher employed distorted perspective grids in several of his prints, including "Up and Down" (1947), "House of Stairs" (1951), and "Print Gallery" (1956). Dick Termes uses a six-point perspective system to paint scenes on spheres and polyhedra, as shown in the example below. The word anamorphic is from
the Greek "ana" (again) and "morphe" (form). It refers to images that are
so heavily distorted that they are hard to recognize without the use of
a mirror, sometimes referred to as an anamorphoscope. When viewed in the
anamorphoscope, the image is "formed again", so that it becomes recognizable.
European painters of the early Renaissance were fascinated by linear anamorphic
images, in which stretched pictures are formed again when viewed on a slant.
A famous example is Hans Holbein's "The Ambassadors" (1533), which contains
a stretched-out skull. The painting could be hung at the top of a stairway
such that people ascending the stairs would be startled by the skull image.
Anamorphic paintings that require cylindrical mirrors were popular in both
Europe and the Orient in the 17th and 18th centuries. These sometimes carried
messages of political protest or erotic imagery. Escher didnít employ conventional
anamorphic grids, though he did employ mirrored spheres in some of his
prints, including his best-known self portrait, "Hand with Reflecting Sphere"
(1935). An example is shown below of a contemporary cylindrical anamorphosis
by István Orosz.
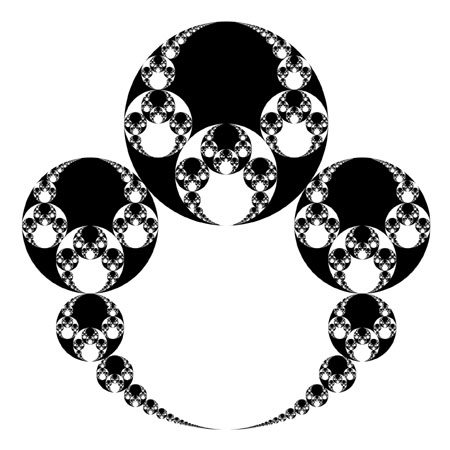
F. Fractals A fractal is an object exhibiting
self similarity on different scales that can be related mathematically.
Fractals are created by iteration, the process of repeatedly carrying out
a calculation or process, so that an object of great complexity and detail
is obtained. Unfortunately, fractals as such were not a tool available
to Escher because they were not formalized and recognized as a distinct
branch of geometry until around the time of his death. Escher was very
interested in depicting the infinite in a finite area, particularly infinite
tessellations. He employed reducing grids and hyperbolic geometry to accomplish
this, as seen in his "Circle Limit" prints I-IV (1958-1960) and "Square
Limit" (1964). Examples are shown below of contemporary works by Kerry
Mitchell and Robert Fathauer.
4. Conclusion Mathematical visual art is
flourishing today, with numerous artists creating works in the tradition
of M. C. Escher and others. These artists work in a wide variety of media,
including sculpture, painting on both flat and three-dimensional "canvases",
print making, and digital art. Themes that are popular with these artists
include polyhedra, tessellations, impossible figures, Möbius bands,
distorted or unusual perspective systems, and fractals. The Exhibit of
Visual Mathematical Art at Bridges presents a cross section of this work.
References [1] M. C. Escher - His Life and Complete Graphic Work, by F.H. Bool, J.R. Kist, J.L. Locher, and F. Wierda (Harry N. Abrams, New York, 1982). [2] The Magic Mirror of M. C. Escher, by Bruno Ernst (Ballantine Books, New York, 1976). [3] Visions of Symmetry - Notebooks, Periodic Drawings, and Related Works of M. C. Escher, by Doris Schattschneider (W.H. Freeman and Co., New York, 1990). [4] "Fractals and an Art for the Sake of Science," Benoit B. Madelbrot, in The Visual Mind, ed. by Michele Emmer (MIT Press, Cambridge, 1993).
|