Isohedrally compatible tilings
Philip M. Maynard |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract: Two of
the 17 (plane) crystallographic groups, G and H, are isohedrally compatible
if there exists a tile which admits separate tilings with respective automorphism
groups G and H. The purpose of this article is to show that of the 136
possible pairs, 77 are definitely compatible, 44 are not and we conjecture
the remaining 15 pairs are also not compatible.
|
1. Introduction to isohedral tilings
Definition: A tiling is called periodic if the automorphism group contains non-parallel translations.
Definition: A tiling is called isohedral if the automorphism group is transitive on tiles. Specifically, if for any two tiles x and y in the tiling there is a symmetry of the tiling which takes x onto y.
Hence in an isohedral tiling there is essentially just one type of tile. An isohedral tiling is necessarily periodic, (this follows, for instance, from the classification of isohedral tilings given in [2]). We now assume that we have a periodic tiling and we wish to establish its automorphism group. It is a surprising fact that there are only 17 different possible automorphism groups arising from periodic tilings, the so-called (plane) crystallographic groups or wallpaper groups. We shall describe a simple procedure which allows us to differentiate among the 17 groups. Imagine a blank transparency covering the tiling and mark on it the following symbols: a small regular n-gon at every centre of rotation of order n (for n = 2 we use a diamond shape), a solid line at each line of reflection, and a dashed line at each line of glide reflection. It is sufficient to do this only on a small portion of the tiling. What you have now is a blueprint for the automorphism group of the tiling. It is now a simple matter of checking this against a list containing all 17 possible blueprints to determine the automorphism group of your tiling. We note that you may need to rotate, re-size or stretch your blueprint to match exactly the ones in the list. We now present the complete list of 17 different blueprints representing the automorphism groups. In practice this procedure can often be done mentally. A more complete discussion of this section can be found in chapter 1 of [2], where essentially we have taken our list, Figure 1. See also [3] for general reference.
Finally, we define compatibility. Let
P and Q be any two of the 17 (plane) crystallographic groups
or wallpaper groups. We call P and Q isohedrally compatible,
or just compatible, if there exists a tile T which admits
both an isohedral tiling whose automorphism group is isomorphic to P
and an isohedral tiling whose automorphism group is isomorphic to Q.
Here we are assuming that the tile T is unmarked. We investigate
this concept in the next two sections.
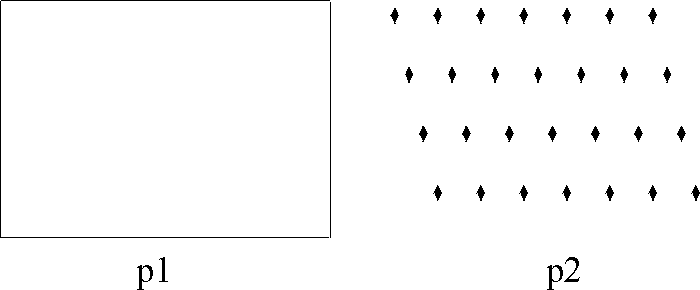

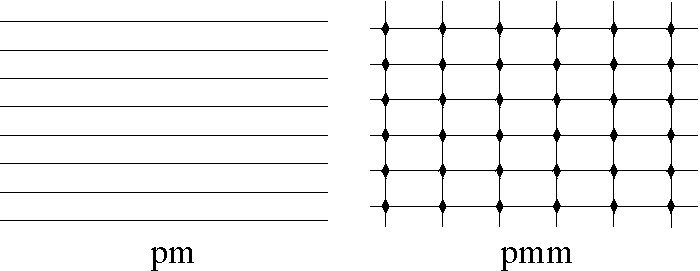
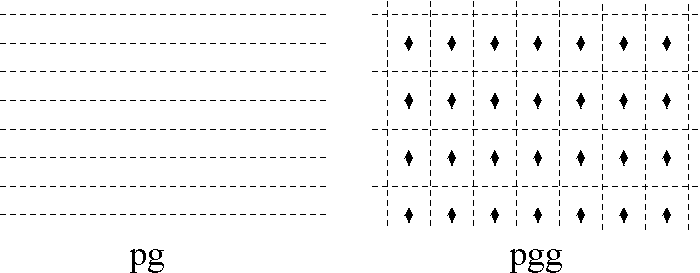
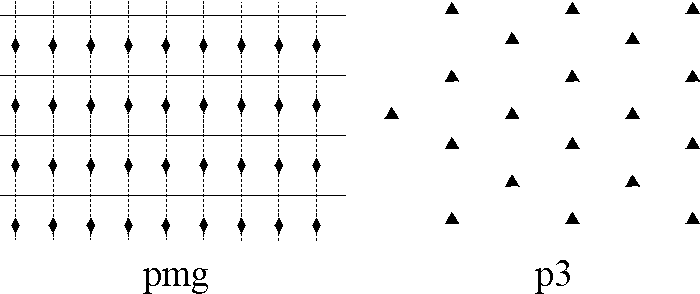
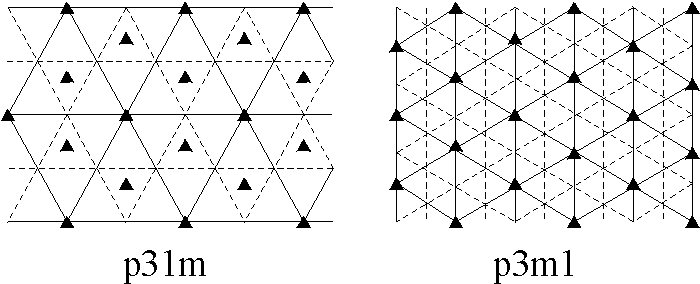
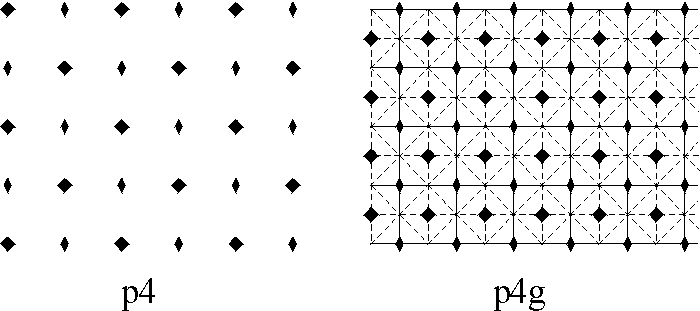
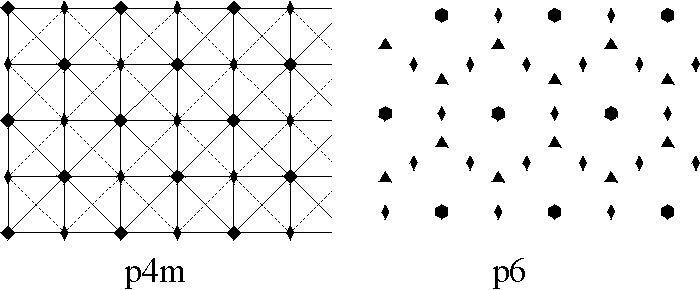
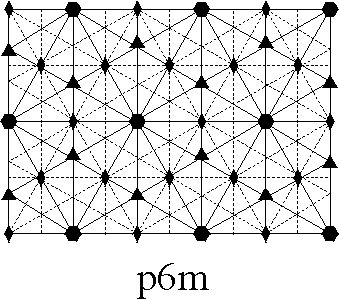
Figure 1: Exhibits all the possible
"blueprints" for
the automorphism group of any periodic tiling.
Example: We present an example
of an isohedral tiling which has automorphism group pgg.
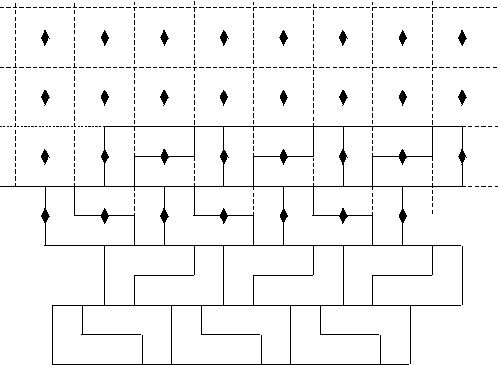
Figure 2: An example of determining the automorphism group of a tiling.
2. Isohedral compatibility: the exceptional cases
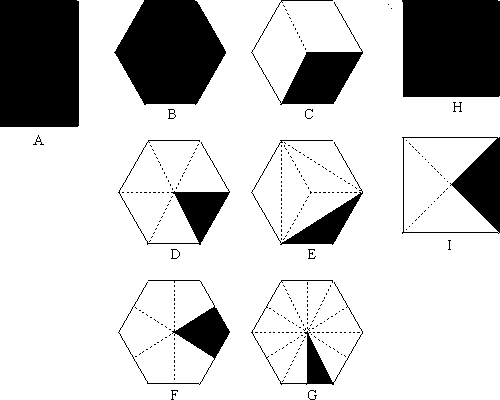
Figure 3: Shows all the possible
tiles (solid) that
admit isohedral tilings with automorphism groups
p4m, pmm or p6m. (A for pmm,
B,
C,
D, E,
F and G for p6m and H and I for
p4m).
The justification of this is as follows. We look at the complete list of unmarked isohedral tiles given in [2] for the above groups and inspect their incidence symbols. In particular we look at IH72, IH76, IH82, IH20, IH32, IH37, IH40, IH77 and IH93. For example, for IH32 we have the incidence symbol [a+a-b+b-;a-b-]. All tilings of the same type are obtained by manipulating the edges using a procedure given in [2, p.279] and by altering the lengths or position of edges. All the incidence symbols we are looking at have the following property: any two edges which abut each other either have the same label but orientated in the opposite direction or have the same label and are unorientated. The procedure in [2] then dictates that for all the incidence symbols we are interested in we must leave the edges as straight lines, i.e., we may only distort the tilings by altering the lengths or positions of edges so long as the symmetries of the tiling are not affected. This is only possible in the case of IH72 and this results in a tiling by rectangles. In all other cases we just "enlarge" or "shrink" our tiling.
We can now determine which of the other
16 crystallographic groups can be isohedrally compatible with the groups
p4m, pmm and p6m. To establish this it is simply a
matter of determining all the possible isohedral tilings using the tiles
in Figure 3. By considering all such possibilities it turns out that it
is sufficient to exhibit all isohedral tilings of the tiles I, A
and C (the other tiles add no new automorphism groups); we show
these tilings in Figure
4, 5 and 6,
respectively.
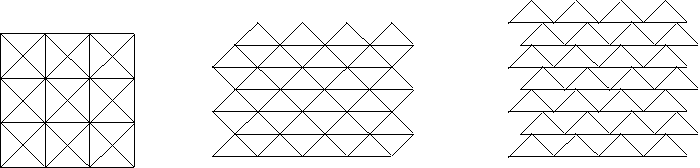
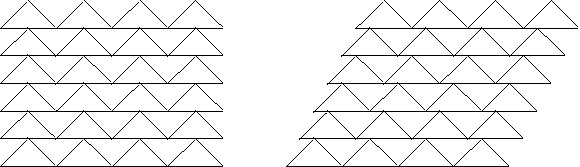
Figure 4: Exhibits all the possible
symmetry groups
with isohedral tilings using the tile I of Figure
3:
p4m, cmm, pgg, pmg, p2.

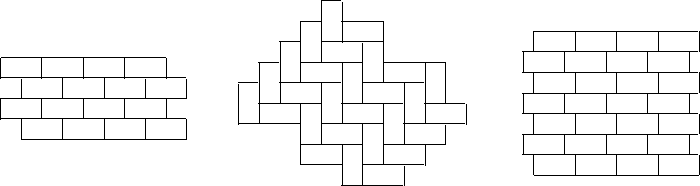
Figure 5: Exhibits all the possible
symmetry groups with
isohedral tilings using the tile A of Figure
3:
pmm, p2, p4g, cmm, pgg, pmg.
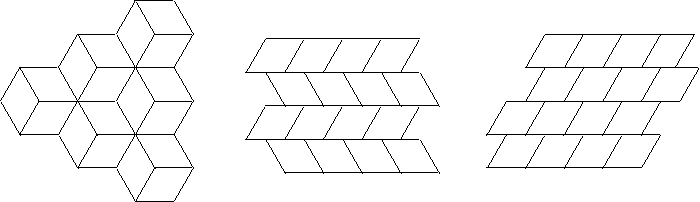
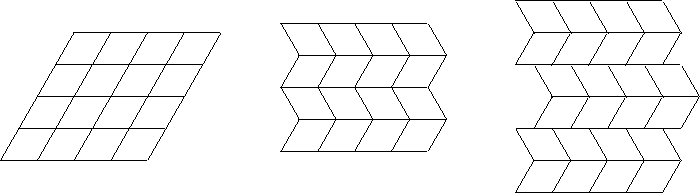
Figure 6: Exhibits all the possible
symmetry groups with
isohedral tilings using the tile C of Figure
3:
p6m, pgg, p2, pmm, pmg, cm.
We summarize the results of this section in a lemma.
Lemma 1. The group p3m1 is not
isohedrally compatible with any of the other 16 crystallographic groups.
Let G1 be isohedrally compatible with p4m then G1
@ cmm, pmg, p2 or pgg. Let G2 be
isohedrally compatible with pmm then G2 @
p2, p4g, cmm, pgg or pmg. Let G3 be isohedrally compatible with
p6m then G3@ pgg, p2, cmm, pmg or
cm.
3. Isohedral compatibility: the general case
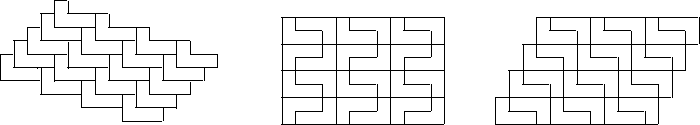

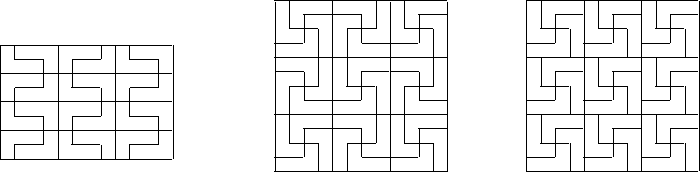
Figure 7: Exhibits isohedral
tilings with the following automorphism groups:
p1, pmg, p2, pg, pgg, cm,
cmm,
p4g,
p4.
We now present tilings with a tile that
admit the following groups of automorphisms: p1, p2, pgg,
cm, pmg,
pm,
pg, (see Figure
8). This gives 6 additional compatible pairs that include pm.

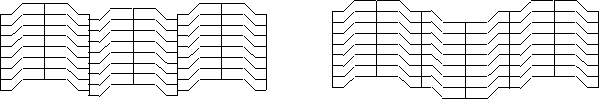

Figure 8: Exhibits isohedral
tilings with the following automorphism groups:
p1, p2, pgg, cm, pmg, pm,
pg.
Figure 9 exhibits tilings with the following
automorphism groups: pg, p2, p1, p3, pgg,
and gives 4 new compatible pairs that include p3.
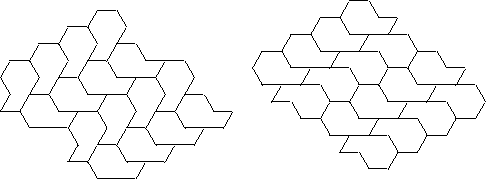
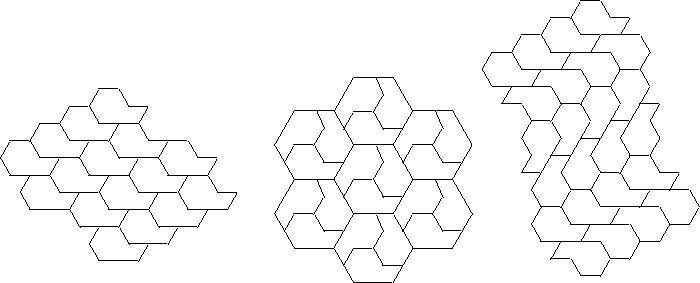
Figure 9: Exhibits isohedral
tilings with the following automorphism groups:
pg, p2, p1, p3, pgg.
The next example, Figure
10, shows a tile that admits 8 different isohedral tilings, and adds
13 new compatible pairs that include
p6 or p31m.
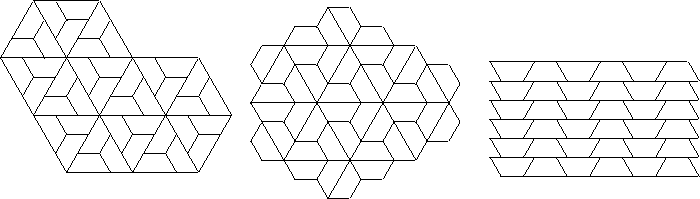

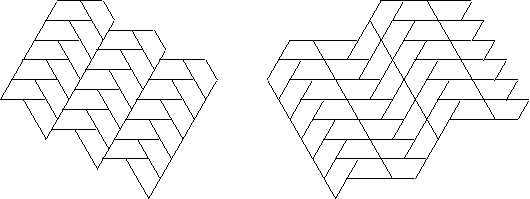
Figure 10: Exhibits isohedral
tilings with the following automorphism groups:
p6, p31m, pmg, cm, p2, cmm,
pg,
pgg.
The tile in the next example has many
different isohedral tilings but we show only the three that give us 2 new
compatible pairs that include p6.
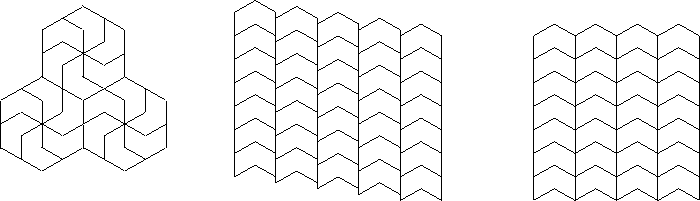
Figure 11: Exhibits isohedral
tilings with the following automorphism groups:
p6, p1,pm.
The next example shows isohedral tilings
with the same tile and automorphism groups p3 and pmg, respectively.
Finally,
Figure 13 shows isohedral tilings admitting
automorphism groups p1 and p31m, respectively. Each of these
examples adds one new compatible pair of groups to our list.
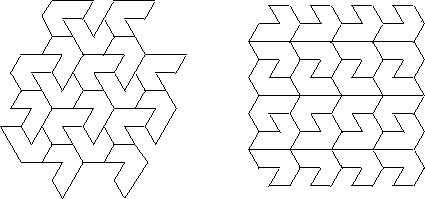
Figure 12: Exhibits isohedral
tilings with the following automorphism groups:
p3, pmg.
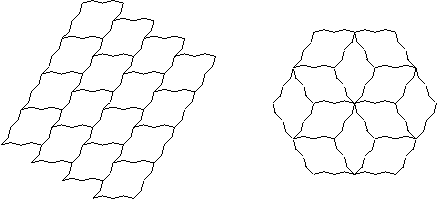
Figure 13: Exhibits isohedral
tilings with the following automorphism groups:
p1, p31m.
We summarize our results in Table
1. A "yes" shows that the two groups are isohedrally compatible and
a question mark means the question is unresolved.
| p1 | pg | p2 | pgg | pmg | cm | pm | cmm | p4 | p4g | p3 | p31m | p6 |
| p1 | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes |
| pg | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes | |
| p2 | yes | yes | yes | yes | yes | yes | yes | yes | yes | yes | ||
| pgg | yes | yes | yes | yes | yes | yes | yes | yes | yes | |||
| pmg | yes | yes | yes | yes | yes | yes | yes | yes | ||||
| cm | yes | yes | yes | yes | ? | yes | yes | |||||
| pm | ? | ? | ? | ? | ? | yes | ||||||
| cmm | yes | yes | ? | yes | yes | |||||||
| p4 | yes | ? | ? | ? | ||||||||
| p4g | ? | ? | ? | |||||||||
| p3 | ? | ? | ||||||||||
| p31m | yes | |||||||||||
| p6 | ||||||||||||
Table 1: Isohedral compatibility between 13 of the crystallographic groups.
4. Remarks
The author has produced many "Escher-type"
symmetry pictures, (isohedral tilings using recognizable shapes for the
tiles). It seemed a natural challenge to produce recognizable tiles, which
can be used in isohedral tilings with 2 (or indeed more) symmetry groups.
The author has produced many such examples (Figs. 14,
15,
16).
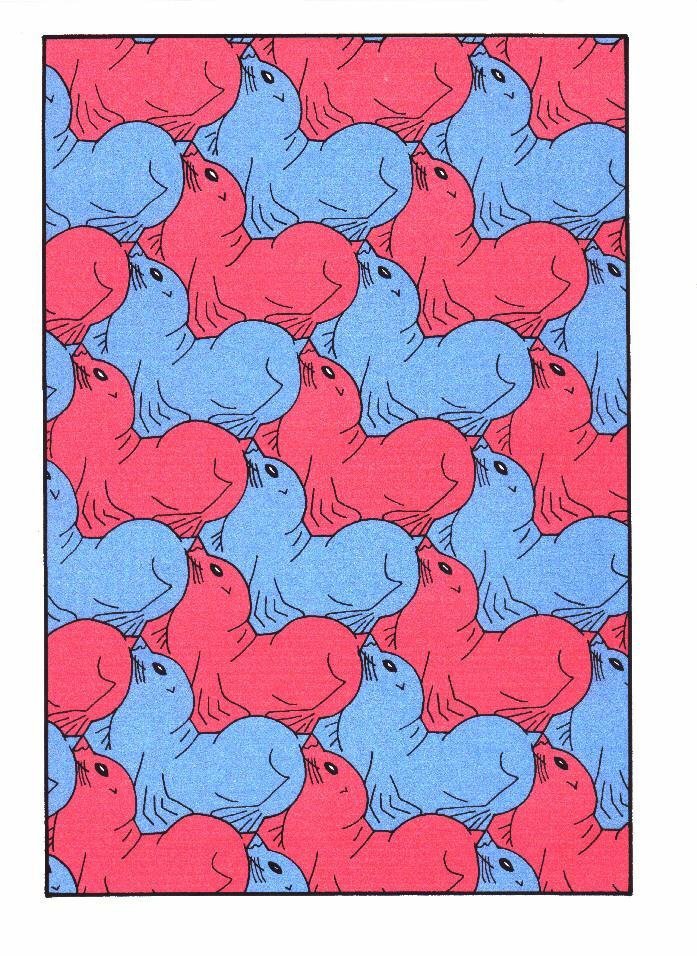
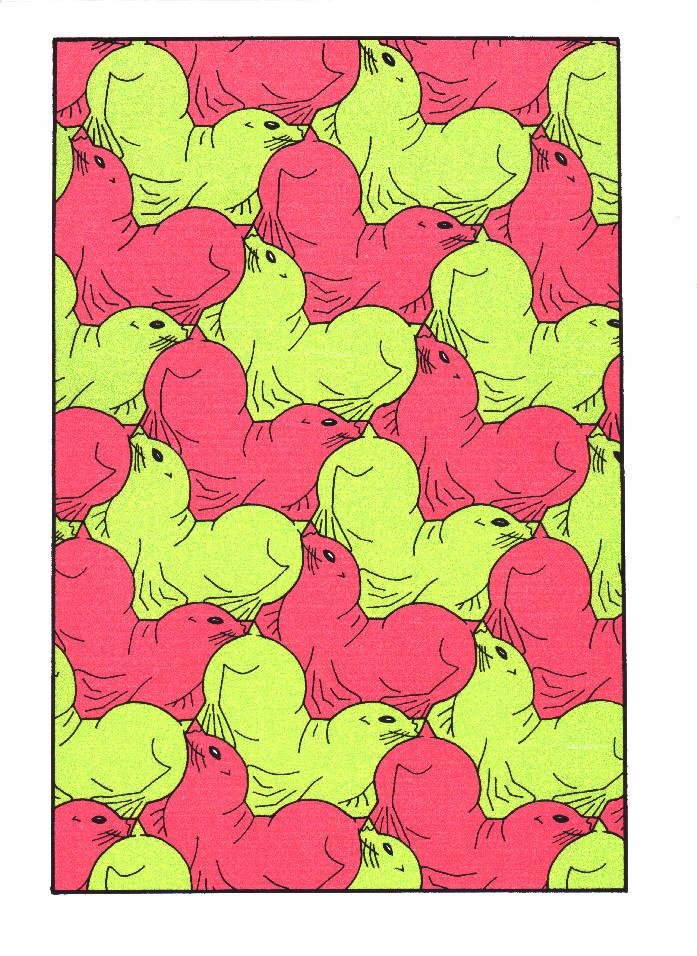
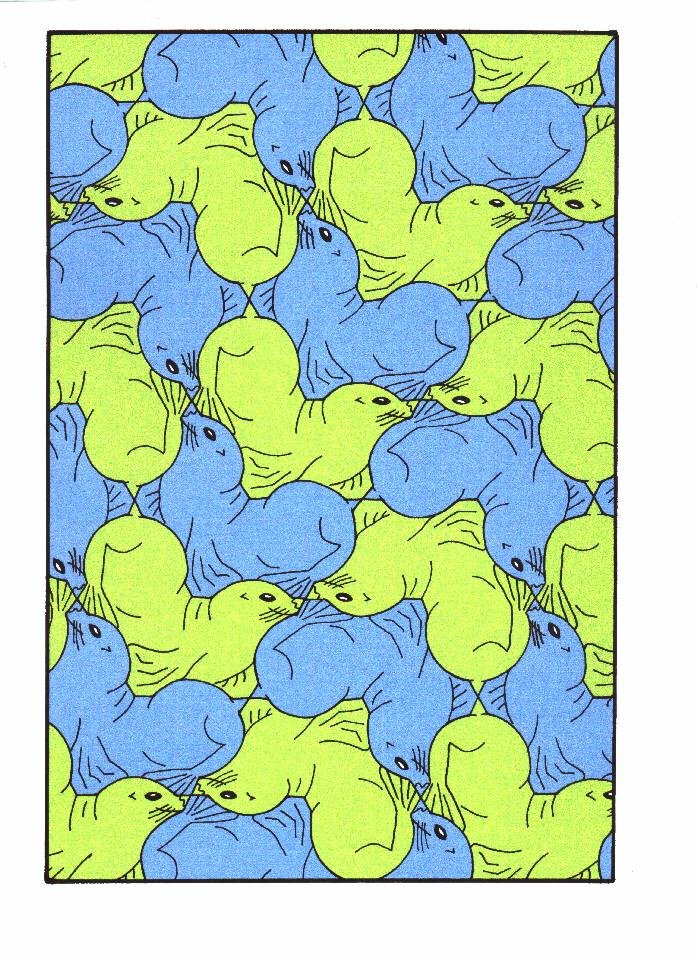
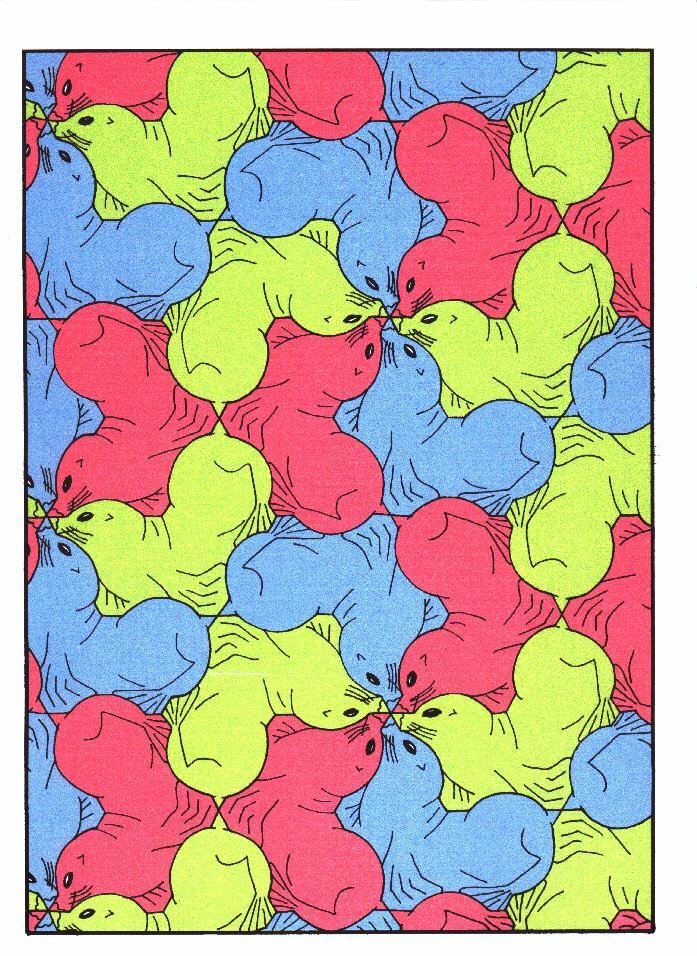
Figure 14: "Sealion", symmetry group p1, pg, pgg, p6.
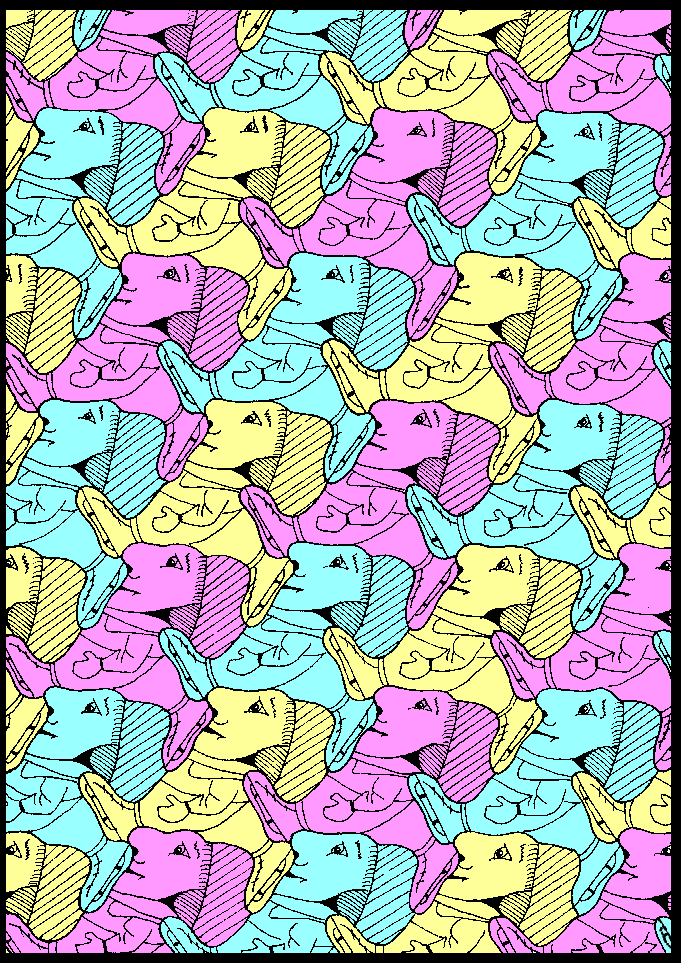
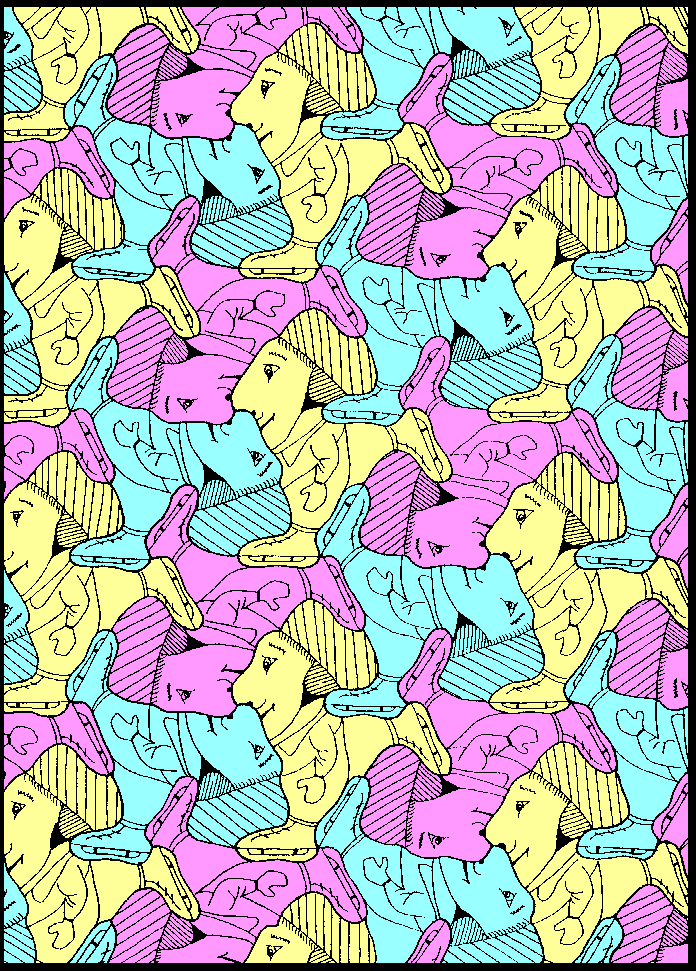
Figure 15: "Skater", symmetry group p1, p3.
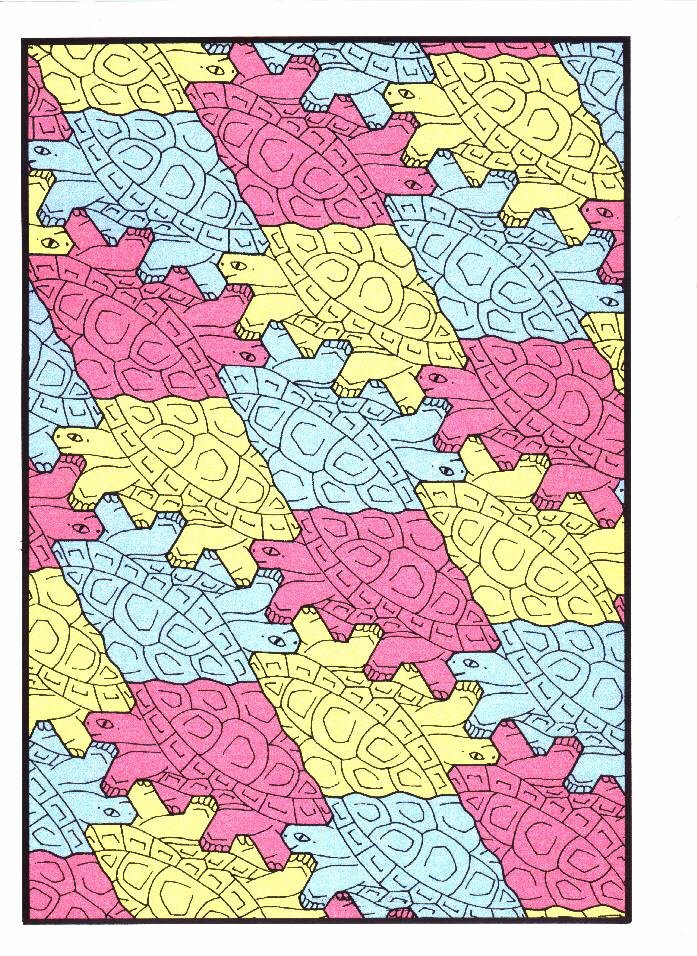
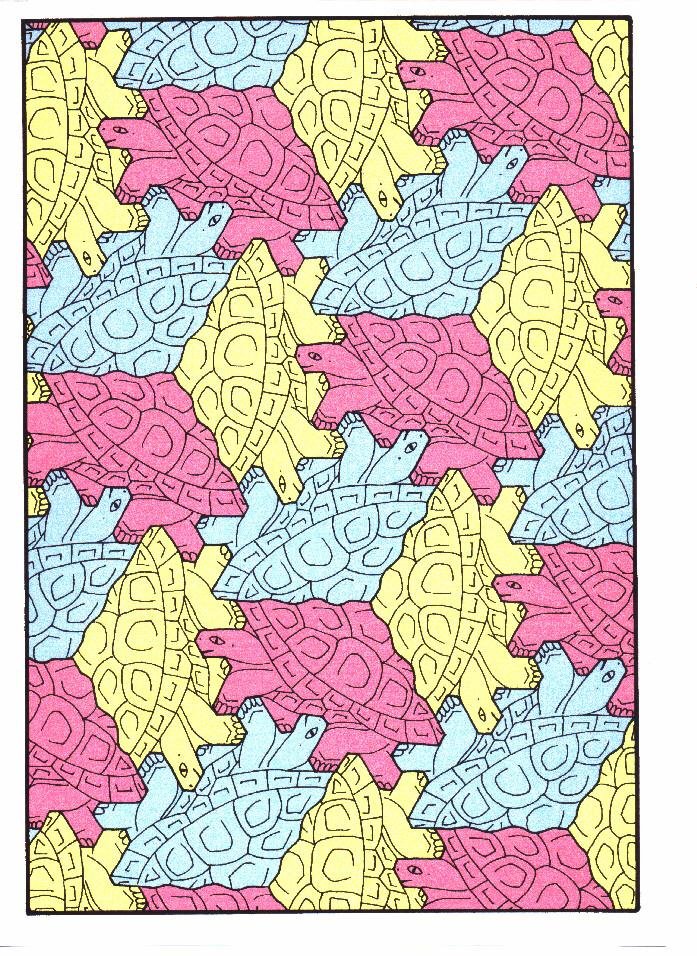
Figure 16: "Tortoise", symmetry group p2, p3.
Acknowledgements
I would like to thank Professor Grünbaum for his helpful correspondence and review of this work.
References
- [1]
- B. Grünbaum and G.C. Shephard (1986), Is there an all-purpose tile? Amer. Math. Monthly 93, 545-551.
- [2]
- B. Grünbaum and G.C. Shephard (1987), Tilings and Patterns, Freeman.
- [3]
- D. Schattschneider (1978), The Plane Symmetry Groups. Their recognition and notation. Amer. Math. Monthly 85, 439-450.
- VisMathHOME