Integration by Parts: a Visual Proof
Sasho Kalajdzievski
Department of Mathematics
University of Manitoba
sasho@cc.UManitoba.CA
1. Introduction.
If ![]() is a Riemann integrable function with respect to
is a Riemann integrable function with respect to ![]() over the interval [a,b] then
over the interval [a,b] then ![]() is Riemann integrable with respect to
is Riemann integrable with respect to ![]() over [a,b], and we have the so called Integration by Parts Formula (in the setting
of Riemann-Stieltjes integrals):
over [a,b], and we have the so called Integration by Parts Formula (in the setting
of Riemann-Stieltjes integrals):
![]() . (*)
. (*)
We assume below that ![]() and
and ![]() have continuous derivatives in [a,b]. Under that assumption, Riemann-Stieltjes
integral reduces to Riemann integral, and (*) can
be interpreted as the more elementary (Riemann) Integration by Parts Formula,
where
have continuous derivatives in [a,b]. Under that assumption, Riemann-Stieltjes
integral reduces to Riemann integral, and (*) can
be interpreted as the more elementary (Riemann) Integration by Parts Formula,
where ![]() stands for
stands for ![]() (and
(and ![]() stands for
stands for ![]() ).We provide a visual justification of the formula (for both Riemann and Riemann-Stieltjes
integrals).
).We provide a visual justification of the formula (for both Riemann and Riemann-Stieltjes
integrals).
2. Proof.
In the pictures we present, both ![]() and
and ![]() are increasing and positive. The other combinations of monotonic functions can
be handled similarly, with due care for the sign of the terms corresponding
to areas of the rectangular regions we use below. Without going into details,
we notice that the general case can be reduced to the preceding by subdividing
the interval [a,b] into subintervals over each of which
are increasing and positive. The other combinations of monotonic functions can
be handled similarly, with due care for the sign of the terms corresponding
to areas of the rectangular regions we use below. Without going into details,
we notice that the general case can be reduced to the preceding by subdividing
the interval [a,b] into subintervals over each of which ![]() and
and ![]() are monotonic (recall that we have assumed continuity of (the derivatives of)
are monotonic (recall that we have assumed continuity of (the derivatives of) ![]() and
and ![]() ).
).
The integral ![]() is the limit of the sum
is the limit of the sum ![]() as
as
![]() tends to infinity and the norm of the partition
tends to infinity and the norm of the partition ![]() of the interval [a,b] tends to 0, taken over all choices of the numbers
of the interval [a,b] tends to 0, taken over all choices of the numbers ![]() .
Note again that under our assumptions on
.
Note again that under our assumptions on ![]() and
and ![]() ,
this definition is compatible with the definition of the Riemann integral (when
,
this definition is compatible with the definition of the Riemann integral (when
![]() is viewed as the Riemann integral
is viewed as the Riemann integral ![]() ).
The sum
).
The sum ![]() can
be interpreted as a sum of areas of the (yellow) vertical rectangles in Picture
1.
can
be interpreted as a sum of areas of the (yellow) vertical rectangles in Picture
1.

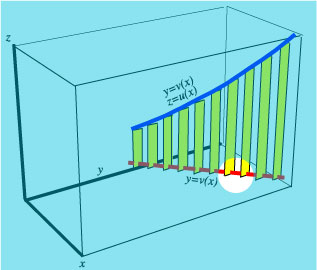
Picture 1.
Zoom in to notice a few features of the image that we will need later.
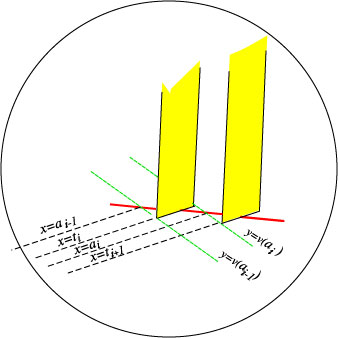
 Picture 2. |
 Picture 3. |
We see in the last image that the (yellow) vertical rectangles are all parallel to the yz-plane and that their orthogonal projections on the yz-plane do not overlap.
Now we do the same visualization of the Riemann/Riemann-Stieltjes
sums corresponding to the integral ![]() .
For reason that will be apparent below, we now interchange the roles of the
y and z axes.
.
For reason that will be apparent below, we now interchange the roles of the
y and z axes.
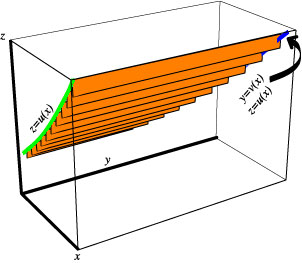
Picture 4.
Here is an amalgamation of the images 1 and 4.
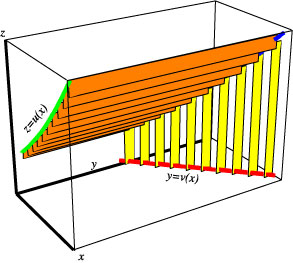
Picture 5.
We rotate this object to get a view of its orthogonal
projection onto the yz-plane. In the animation below we take a finer
subdivision of the interval [a,b] so that the sums ![]() and
and ![]() give better approximations of the integrals
give better approximations of the integrals ![]() and
and ![]() respectively. (Please press and hold the control key and then click on the image
to play the animation or press the right mouse key and then click on the "play" option)
respectively. (Please press and hold the control key and then click on the image
to play the animation or press the right mouse key and then click on the "play" option)
Picture 6 (Animation)
Let us pay attention to the last frame where we see the projection of the rectangles and the curves onto the yz-plane (somewhat off the desired orthogonal projection because of the perspective). As observed above (Picture 3), we do not lose anything of the total area of the rectangles in that projection.
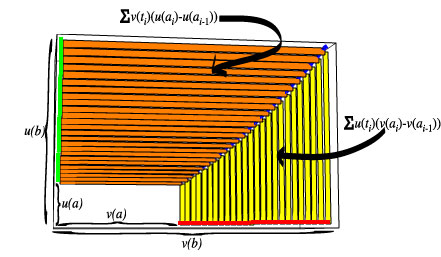
Picture 7.
We see that ![]() +
+ ![]() is
approximately equal to
is
approximately equal to ![]() and (*) follows by taking the limit as
and (*) follows by taking the limit as ![]() .
.