|
Specific properties of symmetries in arts and generalizations of the theory of symmetry An explicit study of the role of symmetry in art begins only with the turn of the century. The reasons for this can be found in the fact that classical esthetics has always been more preoccupied with the interpretation of musical content than with its exact formal analysis, a result of the study of esthetics in modern times which affirms a positivistic and analytic approach in art. On the other hand, for an analysis of this kind to be carried through, a necessary prerequisite is the existence of theoretical assumptions and methods which in the case of the theory of symmetry were formulated in the 19th and 20th centuries. In its implicit form, symmetry is present in all works of art, and it represents a dominant component of all esthetic organizational principles and rules of form. We encounter symmetry already in the acoustic basics of music. M. Ghyka defines noise as a non-periodic acoustic function of time. In order for noise to be formed into music, it must have periodicity which is acquired by rhythmic repetition and a play of harmonics. The sound is simple or perfect when the vibration which forms it is a sinusoid function of time (regular wave movement). In all other cases the sound is complex. Each complex sound is musical if the vibration which engenders it is a periodic function of time (Ghyka, 1987). The subsequent levels of the symmetry organization of music represent the symmetry of the basic musical elements themselves (musical meter and rhythm, tonality, melody, counterpoint, harmony, form, etc). Thus, symmetry appears in music on the level of complete musical forms, as well as in other structural levels. Good illustrations of regularity in music are Bach's fugues based on the complete set of symmetries (basic theme, rectus, inversus, recto-inversus), permutation schemes of bell-ringing used in 17th century England, Schoenberg's 12-tone serial music based on a complete set of 48 sequences derived from the 12-tone system, or the repetition of base motifs and combinatoric manipulation in a given set of tones and rhythms typical of Oriental music (e.g., the Arabic maqa'am system, Hindu raga and tala). A good example of the theory of symmetry applied to musical works is the geometric-symmetry reconstruction made by W. Greitzer in 1926 of the score Kunst der Fuge by J.S.Bach (Nagy, 1990). At first sight, the cited definitions of symmetry may seem sufficiently wide, but they are applicable only to a limited number of works of art. Isometric groups of the symmetry of rosettes, friezes and ornaments apply only to perfectly regular isometric periodic structures such as crystals, or ornaments (plane patterns). Therefore they have a very limited application in the analysis of structural laws in art. Thus, the first and practically the only art discipline which permits symmetry analysis from the aspect of isometric groups of symmetry is ornamental visual art. In other arts is very rare for the same rule of construction to be applied throughout the whole work of art with a uniform repetitive structure as a result. Although one may find examples of such structures in ornamental art, architecture or rhythmic patterns in music, it is much more frequent to come across exceptions to the rules of crystallographic symmetry, resulting in local, as opposed to global symmetries. The global symmetry of work of art is usually present in its form which unites all the other structural levels of the work. On the other structural levels usually dominant are local symmetries (Fig. 1.5).
Here we differentiate between two forms of local symmetries: the appearance of symmetric subparts, or of an element and its image which is acquired by means of a symmetry transformation. An example of local symmetry in music is inversion or retrograde inversion which offers a symmetric subpart, or the repetition of a theme in different parts of the musical piece. One must, however, bear in mind that even in nature there is no perfect symmetry. The seemingly perfect regularity of crystals conceal grains of dislocation. The left side of a human face is not identical to the right side (Deregowski, 1992) (Fig. 1.6). With the increase of the complexity of a certain structure, the different aspects of its symmetry also become more complex. This is particularly true of art works and the possibility of their symmetry analysis. (a)
(b)
It is possible in art to speak about approximate or relative symmetry. In this case equivalent elements are those which are sufficiently common or close to one another, which is why we experience them as equivalent. Thus, when we approach the symmetry analysis of a work of art, we must choose our level of abstraction beforehand, i.e., we must determine the permitted limits of deviation from complete regularity. Because this kind of analysis offers only an approximation of reality, we must previously decide which apparatus of the theory of symmetry we will be using (classical theory of symmetry, antisymmetry, color symmetry, etc.), depending on the characteristics of the study piece. In the generalizations in the field of the theory of symmetry we discern two basic directions:
In the first case, one such dependency may be the constant proportionality of the distance between the points of the original X and Y and the distance between the points of the image t(X)=X1 and t(Y)=Y1, k=X1Y1:XY=const. (Lopandic, 1979). If the coefficient of proportionality k equals 2, we obtain a sequence of proportional line segments XY, 2XY, 4XY, 8XY,... The result of this approach are the transformations of similarity (proportionality, proportional diminishment or augmentation of the figure) (Fig. 1.7). In music, augmentation or diminishing are transformations of similarity (proportionality). The duration of notes is doubled or halved. The next generalization can be obtained in the same manner by introducing non-metric (topological) symmetries.
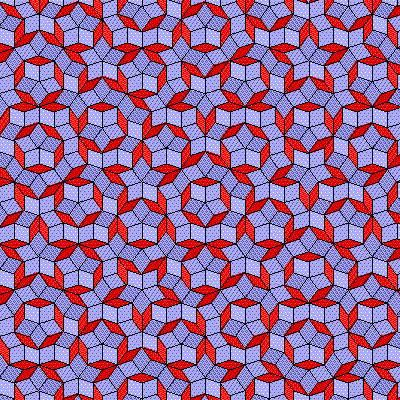
Figure 1.7. Symmetry of similarity. A second type generalization consists of different aspects of colored symmetry - the generalization brought about by the introduction of non-geometrical characteristics and the study of the regular changes of the various qualitative properties (e.g. color) assigned to the system elements. The simplest form of colored symmetry is antisymmetry, bivalent symmetry or the symmetry of opposites (black - white, (+ -), (0 1), rest - duration, major - minor, etc.) (Fig. 1.8)
Figure 1.8. Antisymmetry ornaments of M.C. Escher. A result of these generalizations is the possibility of the analysis of more complex laws of symmetry which are not reducible to mere identical elements and their periodic repetition. Accordingly, a potential object of symmetry analysis can very well be a structure with a lower level of regularity. Unlike strictly regular structures such as isometric crystallographic structures, dynamic laws are applicable here, e.g., the possibility of an analysis of structural changes. Furthermore, with the introduction of the analysis of parameters which indicate a change in quantitative properties (in the sense of colored symmetry), a simultaneous analysis of different symmetries is possible - the study of regular changes of several relevant properties of a piece (rhythm, melody, dynamics, to name a few) and the correlation between them. Besides symmetry, variation and asymmetry are part of every musical piece, which is not the case with regular ornamental structures. The concept of dissymmetry (symmetry breaking) is introduced into modern theory of symmetry with the beginning of the study of partially ordered structures, or OD (order - disorder) structures and biological structures. It is namely the breaking of symmetry that represents a specific property of live matter and dynamic interactive systems, the difference between a crystal and the double helix of a DNA molecule, defined by A. Schroedinger as an "aperiodic crystal." Such an approach to symmetry facilitates the study of real objects and their symmetry, whether the study is about natural symmetry structures (crystals, beehives, the morphological characteristics of living organisms), or the fruits of human activity and the human spirit (works of art). Thanks to the results of the theory of symmetry in the 20th century (theory of antisymmetry, colored symmetry), different aspects of creative art have become accessible to the study of symmetry. Of course, the priority in this area is still given to ornamental art, in the first place in visual arts and architecture, as these forms of art are based on geometric rules accompanied by regular changes in their qualitative properties (e.g., color). Since the study of complex rules that appear in different areas of art requires a very complex mathematical apparatus, the practical application of symmetry analysis in art works is nearly always in reverse proportion to the level of organizational complexity of the work of art that is studied. This is one of the reasons why the area of study in this thesis is mostly limited to simpler musical pieces which can be studied from the aspect of the classical theory of symmetry (isometric symmetry and symmetry of similarity) and which do not require the application of complex generalizations of the theory of symmetry (theory of antisymmetry, colored symmetry, etc.) Another reason is the aspiration to illustrate universal laws of symmetry with basic examples, which can later be applied in a study of more complex structures. The last, but certainly not the least reason is preparing a terrain for the study of the role of symmetry as a factor of auditory perception. In this sense, the thesis explores possible answers to the question: to what extent does the level of regularity of a given musical structure influence its auditory perception, memorization and reproduction? Simpler structures are naturally more appropriate for an experimental study of the role of symmetry.
|