Next: Grundy Number of chocolate
Up: Abstract and the table of contents
Previous: Chocolate games for k = 2
Chocolates that satisfy the equality 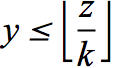 for an arbitrary even number k.
for an arbitrary even number k.
It is easy to generalize the chocolate that satisfies Inequality
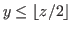 , and we study the chocolate that satisfies the inequality
, and we study the chocolate that satisfies the inequality
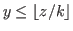 , where
, where
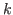 is a natural number.
is a natural number.
We generalize Definition 2.4 and define this chocolate game that satisfies the inequality
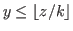 mathematically.
mathematically.
Definition 3.1
There is a black
 block, and on both side of it we have columns of blocks.
The first, the second, the fourth,...,n-th column on the right side of the black block and
block, and on both side of it we have columns of blocks.
The first, the second, the fourth,...,n-th column on the right side of the black block and 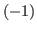 th,
th, 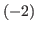 th,...,
th,...,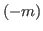 -th column on the left side of the black block.
-th column on the left side of the black block.
The i-th column is
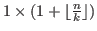 block for
block for
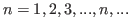 and the i-th column is
and the i-th column is
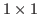 block for
block for
 .
.
Gray blocks are sweet chocolate that can be eaten, and the black block is the bitter chocolate that cannot be eaten.
We define coordinates for this chocolate as in Definition 2.4, and it is easy to see that coordinates 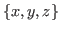 satisfy the inequality
satisfy the inequality
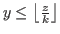 .
.
Next: Grundy Number of chocolate
Up: Abstract and the table of contents
Previous: Chocolate games for k = 2
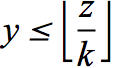 for an arbitrary even number k.
for an arbitrary even number k.
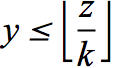 for an arbitrary even number k.
for an arbitrary even number k.
![]() , and we study the chocolate that satisfies the inequality
, and we study the chocolate that satisfies the inequality
![]() , where
, where
![]() is a natural number.
is a natural number.
![]() mathematically.
mathematically.![\includegraphics[height=3.3cm]{k1demo.eps}](img281.png)