Visual Magic Squares and Group Orbits I
John Pais (e-mail) and Richard Singer (e-mail)
©
2004 by John Pais and Richard Singer
“I am
always doing that which I cannot do, in order that I may learn how to do
it.” Pablo Picasso
|
|
|
Abstract
This paper is an essay in visual mathematics that strives to create a guided
discovery in which the learner constructs his/her own math concepts, first
intuitively, exploring, constructing, and counting 4´4 visual magic squares, and second analytically, using
numerical representations and group orbits to classify and generate these
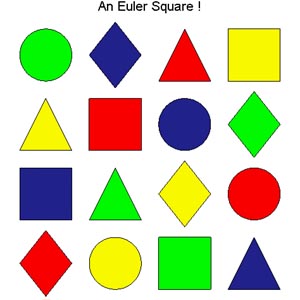
visual magic squares. Euler and Conway visual magic squares are introduced and
used to easily create and identify 528 of the 880 essentially different 4´4 magic squares. Euler items and their
sets of complements are used to count Euler squares. Similarity classes of
The intuitive-analytic duality of the learning experience is commonplace in doing
mathematics (see, e.g. [4]).
Thus, in order to develop this perspective, the reader is guided to the
manifest realization that though a visual representation may be easier to use
to see what is going on intuitively, an appropriate numerical representation
often is necessary to provide an immediate, well-developed theoretical
framework within which one can more easily state and verify important
properties and results. Moreover, this paper is designed to be actively used
for self study and math enrichment with an intended audience including both
secondary school and college level students of mathematics. However, anyone
interested in magic squares should enjoy and benefit from this new visual
approach, presented in the first two sections, which provides the most intuitive
and immediate way to create 4´4 magic
squares that we know. Further, if the reader is familiar with finite groups,
then our new approach using group orbits to generate all
- Euler Squares and Their Representations
- Conway Squares and Their
Representations
- Generating Conway Squares via Group
Orbits
- Acknowledgements and References