Next: Case 5
Up:Abstract and the table of contents Previous: Case 3
Case 4
Next we study the case that 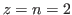
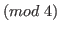 and
and  is odd.
is odd.
An example of this case is Example 5.5.
![\includegraphics[height=10cm]{tablenew1.eps}](img645.png)
In Fig. 5.22 we suppose that Predictions 5.1, 5.2, 5.3,5.4 and 5.5 are valid for
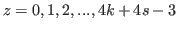 and for
and for
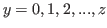 . We also suppose that these predictions are valid for
. We also suppose that these predictions are valid for 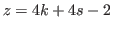 and
and
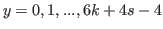 . Under these assumptions we prove that
. Under these assumptions we prove that
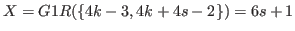 .
.
Remark 5.3
Note that some Grundy numbers
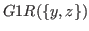 with
with
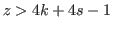 are presented in the table. We do not assume the values of these Grundy numbers to prove this prediction, but the authors present these numbers to show the relative positions of
are presented in the table. We do not assume the values of these Grundy numbers to prove this prediction, but the authors present these numbers to show the relative positions of
 in the table of Grundy numbers.
in the table of Grundy numbers.
Here we assume that
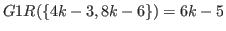 , which is the last number of the sequence
, which is the last number of the sequence
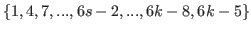 that is an arithmetic sequence with common difference of
that is an arithmetic sequence with common difference of 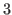 and its length is
and its length is
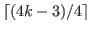 .
.
Therefore the position of
 is in the midst of the the sequence
is in the midst of the the sequence
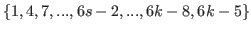 , and this condition make Case 4 very different from Case 1, Case 2 and Case 3.
, and this condition make Case 4 very different from Case 1, Case 2 and Case 3.
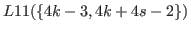
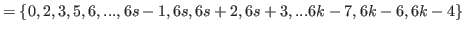
 |
(5.54) |
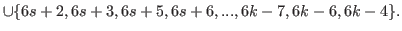 |
(5.55) |
Clearly
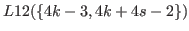
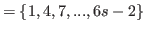 |
(5.56) |
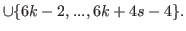 |
(5.57) |
By using Prediction 5.3 for 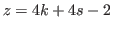 and
and
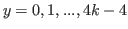
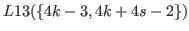
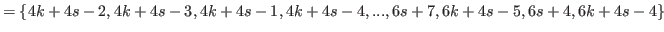
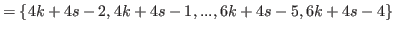 |
(5.58) |
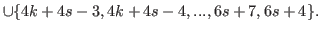 |
(5.59) |
By (5.54) and (5.56) we have
 |
(5.60) |
By (5.55), (5.57), (5.58) and (5.59)
The lists
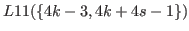 ,
,
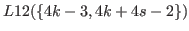 and
and
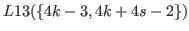 do not contain
do not contain 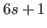 , and hence by
(5.60) we have
, and hence by
(5.60) we have
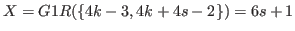 .
.
Next: Case 5
Up:Abstract and the table of contents Previous: Case 3
![\includegraphics[height=10cm]{tablenew1.eps}](img645.png)
![]() ,
,
![]() and
and
![]() do not contain
do not contain ![]() , and hence by
(5.60) we have
, and hence by
(5.60) we have
![]() .
.